Tracking

Depending on the type of tracking, the alignment and inclination angles of the PV modules change during the simulation process. For each time step, the angle of incidence of the sun’s rays is then calculated from the inclination and orientation angles of the PV modules and the position of the sun.

Tracking types

Tracking type	$\alpha_\text{E}$	$\gamma_\text{E}$	Description
None	steady	steady	With permanently mounted modules, inclination and alignment remain constant.
1-axial North-South	steady	variable	The PV modules are oriented to the equator, while the axis of rotation is on the north-south plane. This means that both inclination angle and alignment angle change during the simulation.
1-axial East-West	steady	variable	The PV modules are mounted so that they can rotate around the east-west axis. Here the angle of inclination changes, but not the alignment angle of the modules.
1-axis vertical rotation axis	variable	steady	The PV modules are elevated with a fixed angle of inclination and track the solar azimuth by rotating the vertical axis. This means that the angle of inclination remains constant while the alignment angle changes.
2-axis	variable	variable	The PV modules follow the inclination and orientation of the sun – both angles change in the course of the simulation

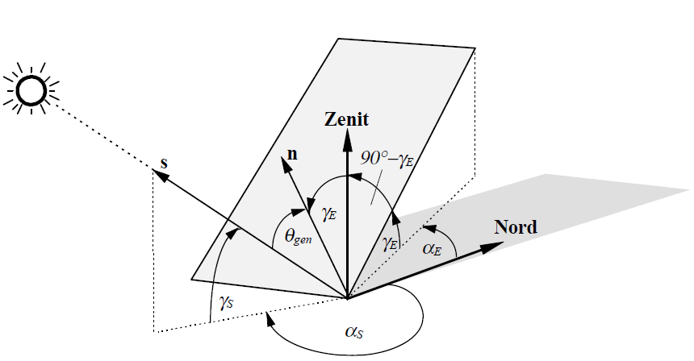
Angle of incidence

The angle of incidence of the radiation on the PV modules $\theta_\text{gen}$ can be calculated from the geometric events.

• $\alpha_\text{S}$: Orientation of the sun
• $\gamma_\text{S}$: Elevation of the sun
• $\alpha_\text{E}$: Orientation of the module
• $\gamma_\text{E}$: Elevation of the module

$\cos(\theta_\text{gen}) = - \cos \gamma_S \cdot \sin \gamma_E \cdot \cos (\alpha_S - \alpha_E + \pi) + \sin \gamma_S \cdot \cos \gamma_E$

Source of graphic: Quaschning: “Simulation der Abschattungsverluste bei solarelektrischen Systemen” pdf